Have you ever wondered about the fascinating world of Trigonometry? Well, look no further! In this article, we will explore the basics of Trigonometry, how it relates to other mathematical concepts such as Algebra and Geometry, and how it can be applied in real-life situations. Whether you are a student studying for your GCSE Maths exam, a teacher looking for useful resources, or simply someone curious about the subject, this introduction to Trigonometry will provide you with a solid foundation to start your journey into this exciting field of mathematics. So, grab your calculator and let’s embark on this mathematical adventure together!
What is Trigonometry?
Trigonometry is a branch of mathematics that deals with the relationships between the angles and sides of triangles. It encompasses various functions and concepts that are used to solve problems related to angles and distances. Trigonometry finds its applications in a wide range of fields, including engineering, physics, architecture, astronomy, and more. In this article, we will dive into the definition, history, functions, applications, and problem-solving techniques of trigonometry.
Definition of Trigonometry
Trigonometry can be defined as the study of the relationships between angles and sides of triangles. It involves the use of trigonometric functions, such as sine, cosine, tangent, cosecant, secant, and cotangent, to analyze and solve mathematical problems. Trigonometry helps us understand the properties and behavior of triangles, enabling us to measure distances, determine angles, and solve various real-life problems.
History of Trigonometry
Trigonometry has a rich and fascinating history that dates back to ancient civilizations. The ancient Egyptians and Babylonians were among the first to document methods for measuring angles and constructing right-angled triangles. However, it was the ancient Greeks who laid the foundation for trigonometry as a mathematical discipline. Mathematicians like Hipparchus and Ptolemy made significant contributions in developing trigonometric tables and formulas. Trigonometry further evolved during the Islamic Golden Age, with scholars like Al-Khwarizmi and Al-Battani making significant advancements. Today, trigonometry continues to evolve and be utilized in various fields of study.
Trigonometric Functions
Trigonometric functions are the basic building blocks of trigonometry. They are mathematical functions that relate the angles and sides of a triangle. Understanding these functions is essential in solving trigonometric problems. Let’s take a look at the main trigonometric functions:
Sine
The sine function (sin) relates the ratio of the length of the side opposite an angle to the hypotenuse of a right-angled triangle. It is defined as the ratio of the length of the side opposite an angle to the hypotenuse.
Cosine
The cosine function (cos) relates the ratio of the length of the adjacent side to the hypotenuse of a right-angled triangle. It is defined as the ratio of the length of the adjacent side to the hypotenuse.
Tangent
The tangent function (tan) relates the ratio of the length of the side opposite an angle to the length of the adjacent side of a right-angled triangle. It is defined as the ratio of the length of the side opposite an angle to the length of the adjacent side.
Cosecant
The cosecant function (csc) is the reciprocal of the sine function. It is defined as the reciprocal of the sine of an angle.
Secant
The secant function (sec) is the reciprocal of the cosine function. It is defined as the reciprocal of the cosine of an angle.
Cotangent
The cotangent function (cot) is the reciprocal of the tangent function. It is defined as the reciprocal of the tangent of an angle.
Relationship between Trigonometric Functions
The trigonometric functions are interrelated and have various identities and formulas that help establish relationships between them. These relationships are fundamental in solving trigonometric equations and manipulating trigonometric expressions. Understanding the relationships between the functions allows us to simplify expressions, perform calculations, and solve complex problems.
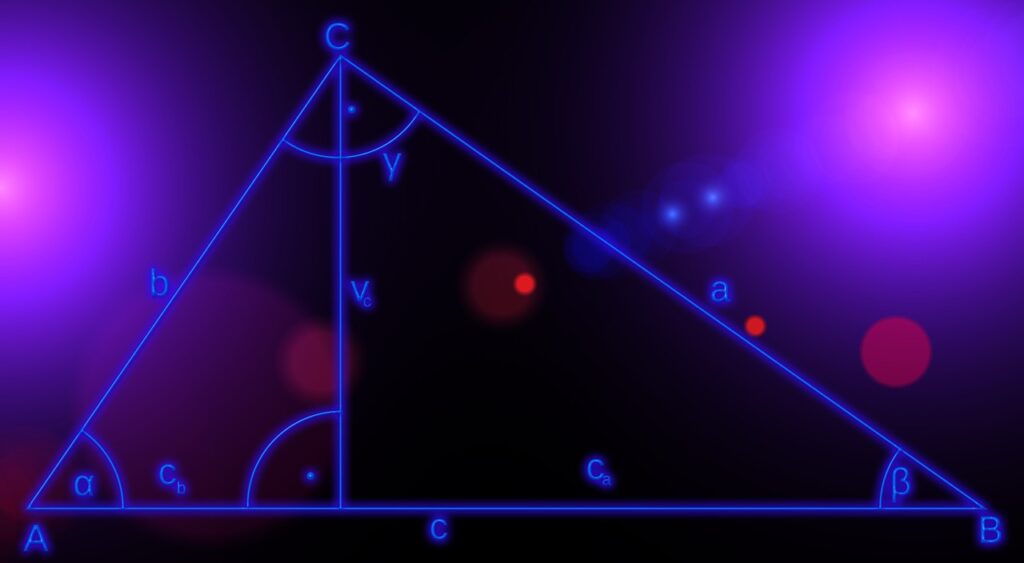
Right-angled Triangles
A right-angled triangle is a triangle that contains a right angle (90 degrees). Right-angled triangles play a significant role in trigonometry, as they provide a standardized shape for analyzing angles and sides. Understanding the properties and relationships within right-angled triangles is crucial in applying trigonometric principles. Let’s explore some key aspects of right-angled triangles:
Definition of a Right-angled Triangle
A right-angled triangle is a triangle that has one angle measuring 90 degrees. The side opposite the right angle is called the hypotenuse, while the other two sides are known as the legs. The properties and ratios within a right-angled triangle form the foundation of trigonometry.
Pythagorean Theorem
The Pythagorean theorem is a fundamental principle in trigonometry that relates the lengths of the sides of a right-angled triangle. It states that the square of the hypotenuse is equal to the sum of the squares of the other two sides. The Pythagorean theorem can be expressed as follows: c^2 = a^2 + b^2, where c represents the length of the hypotenuse, and a and b represent the lengths of the other two sides.
Trig Ratios in Right-angled Triangles
Trig ratios, such as sine, cosine, and tangent, can be applied to right-angled triangles to determine the relationships between angles and sides. These ratios help in finding the unknown angles or side lengths of a triangle. By using trigonometric functions and trigonometric identities, we can calculate the values of various angles and sides within a right-angled triangle.
Solving Right-angled Triangles
Solving right-angled triangles involves finding the values of all angles and sides given specific information about the triangle. This information could include the length of one side and the measurement of an angle, or the lengths of two sides. By utilizing trigonometric functions, the Pythagorean theorem, and other trigonometric identities, we can determine the values of missing angles and sides within the triangle.
Unit Circle
The unit circle is a fundamental concept in trigonometry that relates angles to the coordinates on a circle. It provides a valuable tool for visualizing and understanding trigonometric functions. Let’s delve into the key aspects of the unit circle:
Definition of a Unit Circle
A unit circle is a circle with a radius of 1 unit, centered at the origin of a coordinate system. It is often used as a reference for measuring and understanding angles in trigonometry. By examining the relationship between the trigonometric functions and the coordinates on the unit circle, we can derive the values of these functions for any given angle.
Radians and Degrees
Angles can be measured in both degrees and radians. While degrees are widely used in everyday life, radians are the preferred unit of measurement in trigonometry. A radian is defined as the measure of an angle that subtends an arc equal to the length of the radius of a circle. The conversion between radians and degrees is crucial in trigonometric calculations.
Trigonometric Functions on the Unit Circle
The unit circle provides a valuable reference for calculating the values of trigonometric functions for any given angle. By associating the coordinates on the unit circle with the trigonometric functions, we can determine the values of sine, cosine, tangent, and other trigonometric functions. This association leads to the establishment of the unit circle as a comprehensive tool for understanding and applying trigonometry.
Graphs of Trigonometric Functions
The graphs of trigonometric functions provide visual representations of how these functions behave as the angle changes. Each trigonometric function has a unique graph with specific characteristics. By analyzing these graphs, we can gain insights into the behavior, periodicity, and amplitude of the trigonometric functions. Graphs of trigonometric functions are invaluable in solving trigonometric equations and understanding the properties of these functions.
Trigonometric Identities
Trigonometric identities are equations that relate different trigonometric functions. These identities provide valuable tools for simplifying expressions, manipulating equations, and establishing relationships between various trigonometric functions. Let’s explore the key aspects of trigonometric identities:
Definition of Trigonometric Identities
Trigonometric identities are equations that hold true for all values of the variables within the domain of the functions involved. These identities establish relationships between different trigonometric functions, angles, and sides of triangles. Trigonometric identities play a crucial role in solving equations, simplifying expressions, and establishing connections between various trigonometric concepts.
Fundamental Trigonometric Identities
The fundamental trigonometric identities are the building blocks of trigonometry. They form the basis for establishing relationships between the trigonometric functions. These identities include the reciprocal, quotient, and Pythagorean identities. By utilizing these identities, we can simplify complex expressions, perform calculations, and solve trigonometric equations.
Sum and Difference Formulas
The sum and difference formulas are used to calculate the trigonometric functions of the sum or difference of two angles. These formulas allow us to express trigonometric functions in terms of other trigonometric functions. By applying these formulas, we can simplify trigonometric equations, manipulate trigonometric expressions, and establish relationships between angles and functions.
Double Angle Formulas
Double angle formulas enable us to express trigonometric functions in terms of twice the angle or half the angle. These formulas are derived from the sum and difference formulas and are useful in simplifying trigonometric expressions and solving trigonometric equations. By utilizing the double angle formulas, we can relate the trigonometric functions of a given angle to those of a doubled or halved angle.
Half Angle Formulas
The half-angle formulas allow us to express trigonometric functions in terms of half the angle. These formulas are derived from the double angle formulas and provide a method for replacing a trigonometric function of an angle with another trigonometric function of half that angle. Half-angle formulas are valuable in simplifying expressions and solving trigonometric equations.
Trigonometric Equations
Trigonometric equations involve the unknown values of trigonometric functions. Solving these equations requires manipulating the trigonometric expressions and applying various identities and formulas. Let’s explore the key aspects of trigonometric equations:
Solving Trigonometric Equations
Solving trigonometric equations involves finding the values of the unknown angles or sides that satisfy the given equation. This process requires applying trigonometric identities, formulas, and solving techniques to simplify and solve the equation. By utilizing the properties of trigonometric functions and employing algebraic manipulation, we can derive the solutions to trigonometric equations.
General Solutions and Special Solutions
Trigonometric equations often have infinitely many solutions, known as general solutions. These solutions can be expressed in terms of integers or variables. Special solutions, on the other hand, are specific values within the general solution that satisfy the equation. By identifying the special solutions, we can determine the specific solutions to a trigonometric equation in a given range or interval.
Trigonometry in Real-Life Applications
Trigonometry finds numerous applications in various fields, both practical and theoretical. Its principles and concepts are utilized in solving real-life problems and understanding the physical world around us. Let’s explore some key applications of trigonometry:
Applications in Engineering
Trigonometry plays a vital role in engineering, where it is used in designing and analyzing structures, calculating forces and angles, surveying land, and navigating the terrain. Engineering disciplines such as civil, mechanical, electrical, and aerospace heavily rely on the principles of trigonometry to ensure the safety, accuracy, and efficiency of their designs and calculations.
Applications in Physics
Trigonometry is a fundamental tool in physics, enabling the measurement and analysis of various physical phenomena. It is used in analyzing trajectories, calculating distances, determining velocities, finding forces, and understanding the behavior of waves and vibrations. Trigonometry provides a mathematical framework for modeling and understanding the fundamental laws and principles of physics.
Applications in Architecture
Trigonometry is essential in architecture, where it is used to design and construct buildings, bridges, and other structures. Architects rely on trigonometry to calculate angles, determine the dimensions and proportions of structures, and ensure their stability and functionality. Trigonometry helps architects transform their creative visions into tangible and structurally sound designs.
Applications in Astronomy
Trigonometry plays a critical role in astronomy, where it is used to study the movement, distances, and properties of celestial bodies. By applying trigonometric principles, astronomers can determine the positions and distances of celestial objects, calculate the sizes and scales of celestial bodies, and model their motion and interactions. Trigonometry enables us to explore and understand the vastness of the universe.
Trigonometry in Problem Solving
Trigonometry offers powerful problem-solving techniques that can be applied to various real-life situations. By utilizing trigonometric functions, identities, and formulas, we can analyze and solve complex problems efficiently. Let’s explore some key aspects of trigonometry in problem-solving:
Using Trigonometry to Solve Real-Life Problems
Trigonometry can be used to solve a wide range of real-life problems, such as finding heights and distances, calculating angles, analyzing trajectories, and determining velocities. By employing trigonometric principles, we can apply mathematical concepts to real-world situations and solve problems involving angles, distances, and other geometric properties.
Applications in Navigation and Surveying
Trigonometry is vital in navigation and surveying, where it is used to determine positions, distances, and directions. Navigators and surveyors rely on trigonometric concepts to plot courses, calculate distances between points, and determine the angles and heights of landforms. By utilizing trigonometry, precise navigation and accurate measurements can be achieved in various land and sea-based applications.
Trigonometry in Geometry
Trigonometry has significant applications in geometry, where it is used to prove theorems, find unknown angles and side lengths, and analyze geometric relationships. Let’s explore some key applications of trigonometry in geometry:
Applications in Geometric Proofs
Trigonometry is often employed in geometric proofs, where it helps establish relationships between angles, sides, and geometric objects. By utilizing trigonometric identities and formulas, geometric proofs can be simplified and logical connections between various elements can be established. Trigonometry provides a mathematical framework for proving and understanding geometric properties and theorems.
Finding Unknown Angles and Side Lengths
Trigonometry is instrumental in finding unknown angles and side lengths in geometric figures. By applying trigonometric functions and ratios, we can determine the values of angles and side lengths in triangles, quadrilaterals, and other polygons. Trigonometry allows us to explore and calculate the properties of geometric shapes, helping us solve geometric problems and establish geometric relationships.
Trigonometry in Calculus
Trigonometry is closely linked to calculus, as it provides the necessary tools and concepts for solving trigonometric integrals and derivatives. Let’s explore some key applications of trigonometry in calculus:
Applications of Trigonometry in Calculus
Trigonometry is utilized in calculus to solve problems involving trigonometric functions and their derivatives or integrals. Calculus allows us to analyze the rate of change of trigonometric functions, determine the maximum and minimum values, and calculate areas bounded by trigonometric curves. Trigonometry provides the foundation for tackling more complex calculus problems involving trigonometric functions.
Trigonometric Substitutions
Trigonometric substitutions are mathematical techniques used in calculus to simplify integrals involving trigonometric functions. By making appropriate substitutions based on trigonometric identities, complex integrals can be transformed into simpler forms that are easier to solve. Trigonometric substitutions enable us to work with familiar trigonometric functions and manipulate integrals effectively.
In conclusion, trigonometry is a versatile branch of mathematics that finds applications across various fields and disciplines. Through its functions, identities, and concepts, trigonometry enables us to analyze and solve problems related to angles, triangles, and real-life scenarios. Whether it’s engineering, physics, architecture, or countless other applications, trigonometry plays a vital role in understanding and navigating our world. By mastering the principles and techniques of trigonometry, we can unlock new perspectives and problem-solving skills that have wide-ranging practical and theoretical implications.